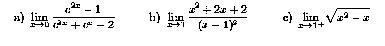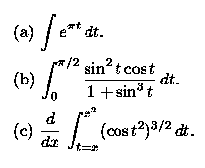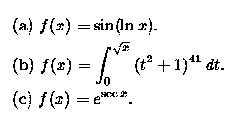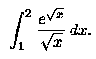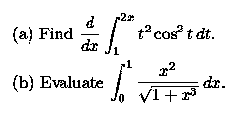Mathematics 140

Chapter 2

• Review Worksheet for Chapter 2

  The following problems have been taken from Math 140 exams of the last few years.
  1. 1. Complete the following definition of a limit. Let f be a function defined on an open interval containing a, except possibly at a. Then L is the limit of f(x) as x approaches a if ....
     2. Using the notation of the above definition, let f(x) = x² + 2x + 3, a = 10 (so that L = 123), and suppose epsilon = 0.1. Use your calculator to find a delta which satisfies the condition(s) of the above definition. Sketch the graph of f on an appropriate interval to show that your delta works.
  2. For the function f below, determine if f is continuous at a, continuous from the right at a, or continuous from the left at a, for a = 0 and a = 1: f(x) = (sin x) / x for x < 0,
     x² + e^x for 0 <= x <= 1,
     x + 1 for x > 1.
  3. Determine which of the following limits exist. If the limit exists, then evaluate it. This is not a calculator problem; you must provide some algebra and explanation.
     1. lim as u -> 0 [e^3u - e⁰]/[2u]
     2. lim as t -> 0 [sqrt t + tan (2 sqrt t]/(sqrt t)
     3. lim as z -> 0 [1/ ln z]
  4. 1. Show that the equation x³ - x = -2 has at least one solution. This is not a calculator problem, you must quote the facts you are using and verify that they apply to this problem.
     2. Now use your calculator to locate all the solutions numerically (to two decimal places).

• Math 140 First Hourly Exam, September 19, 1997

  This is a closed book, closed notes 50 minute exam. You may use your calculator. Do one numbered problem on each of the four answer sheets. Be sure your name and your TA's name and the problem number are on each page. Show all your work. If the grader cannot tell how you obtained an answer you will not receive full credit, even if the answer is correct.
  1. a) (5 points) Solve the following equation for x: ln (x²-1) - 2 ln (x+1) = ln 2. b) (5 points) Let l be the line with equation 2x + 4y = 1. Find an equation for the line perpendicular to l and passing through the point P = (-1,2).
     c) (10 points) Compute the value of sin [pi/3 + pi/4].
  2. a) (5 points) The height of an oscillating weight at time t is given by the function h(t) = e^t/2 sin(2t). State the limit you must evaluate in order to find the vertical velocity of the weight at time t = 0.
     b) (10 points) Evaluate this limit.
     c) (10 points) Use the Squeezing Theorem to find the limit Give your choice for the lower function f and the upper function h. What inequalities are you using?
  3. (30 points) State which of the following limits exist as numbers or as infinity or -infinity and why. Evaluate those that exist.
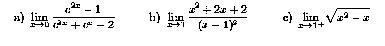
  4. a) (10 points) State the definition of what it means for a function f to be continuous on the closed interval [a, b].
     b) (15 points) In each of the following three intervals [-3, -1], [-1, 1], and [1, 3] determine whether or not the Intermediate Value Theorem guarantees the existence of a zero of the function f defined by f(x) = x - 3 + 1 / x. Justify all your answers.

Chapter 3

• Math 140 Second Hourly Exam, October 10, 1995

  This is a closed book, closed notes 50 minute exam. You will need to use your calculator. If a problem does not ask you to use your calculator, we do not expect you to use it in solving the problem. Do one numbered problem on each of the four answer sheets. Be sure your name and your TA's name and the problem number are on each page. Show all your work. If the grader cannot tell how you obtained an answer you may not receive any credit, even if the answer is correct.
  1. (10 points) Suppose f is a function differentiable at a value a. Write an equation for the tangent line to the graph of f at (a, f(a)) in terms of f and f'.
     b) (10 points) Approximate the cube root of 28 using differentials.
     
  2. (30 points) Find the derivatives of the following functions: (a) (cube root of x) + x cos x
     (b) square root of (2 + sin² x)
     (c) (2^x) / (1 + 3^x).
  3. (10 points) Suppose x and y satisfy e^xy= y. Find dy / dx by implicit differentiation.
     b) (10 points) Use the Newton-Raphson Method and your calculator to find an approximate solution for x³ = 28. Continue until two successive approximations on your calculator agree. Use 3 as initial seed value and state the function you use to calculate the successive approximations. List all the successive approximations you calculate putting a box around the last one.
  4. (30 points) Joan is holding the string of a kite. John stands fifteen feet from Joan and releases the kite. It rises vertically into the air. Joan lets out the string at a constant rate of 10 feet per second. At what rate is the kite rising vertically when it is twenty feet off the ground?

• Review Worksheet for Chapter 3

  The following problems have been taken from Math 140 exams of the last few years.
  1. a. Let f(x) = x / (x+1). Use the definition of the derivative as a limit to find f'(1).
     b. Let f(x) = x² + sin x - 1. Use the Newton-Raphson method and your calculator to approximate a solution in (0, 1) of f(x) = 0. Continue until the output of your calculator does not change. Write down your initial value and the final output of the calculator.
     c. Let g(x) = x³ - x - 2. Using the seed value x1 = 1 in the Newton-Raphson method, find the next two approximates x2 and x3 for a root of g(x).
  2. a. In the following, find f'(x). (i) f(x) = (tan x) / (-3 + sqrt x)
     (ii) f(x) = (3x - 2 sin x)^5/2. b. Suppose y is a function of x that satisfies x⁵ cos y + 3x - y² = 1. Find dy / dx.
     c. Let f(x) = x sec x. Find f''(x).
  3. a. Let f(x) = x - 1 / x. Find an equation of the line tangent to the graph of f at (1, 0).
     b. A right triangle whose two legs always have the same length is growing in size. Its area is increasing at a rate of 100 square miles per year. How fast is the length of the hypotenuse of the triangle increasing when the area is sqrt 2 square miles?
     c. Water is leaking onto a floor from a dripping faucet. The water is forming a semi-circular puddle next to a wall. The puddle is growing in surface area at a rate of 10 square inches per minute. How rapidly is the radius of the puddle growing at the moment when the area of the puddle is 50 pi square inches?

Chapter 4

• exam from fall 1997

Chapter 5

• exam from fall 1997

The Whole Course

• Final Exam, May 1997

  1. Find the derivatives of
     1. tan (3x) / (1 + x³).
     2. ln ( sin (x² + x + 1)).
  2. Find an equation of the line tangent to the graph of x⁴ + y⁴ = 17 at the point (2,1).
  3. Compute the indicated limits:
     1. limit as x goes to 0 of (x / tan (19x)).
     2. limit as x goes to infinity of ((3x² + 5) / (4x² - x + 1)) + ((sin x) / x )
  4. Assume that the half-life of radium is 1590 years. How long will it take for 99% of a sample of 100 grams to disappear?
  5. 1. Show that the equation x⁵ + 1 = x² + 4 has a solution which lies between 0 and 2. Carefully explain how this follows from the appropriate theorem.
     2. Use Newton's Method to estimate this solution to as many places as your calculator allows. Specify your first guess and the first iterate as well as the final answer.
  6. A cylindrical barrel (with a circular base) is to contain 32 cubic meters. Suppose that material for the side costs $1 per square meter and the (different) material for the top and bottom costs $2 per square meter. Find the dimensions of the barrel for which the cost of the material is least.
  7. Let f (x) = (x - 3) / (x - 2)². You may assume that f' (x) = (4 - x) / (x - 2)³,
     f'' (x) = 2 (x - 5) / (x - 2)⁴.
     1. Determine the intervals on which f is increasing, and the intervals on which f is decreasing.
     2. Determine all relative extreme values of f, and where they occur.
     3. Determine the intervals on which f is concave downward, and the intervals on which f is concave upward.
     4. Find all inflection points of f.
     5. Determine all asymptotes of f.
     6. Carefully sketch the graph of f, incorporating the information from above. Note: you may use your calculator here, but no credit will be given for a sketch without the requested discussion.
  8. 1. Let f (x) = x². Using the definition of the derivative as a limit, prove that f' (10) = 20.
     2. Let f (x) = 1 - x⁴. Compute the upper sum for the partition P = {-2, -1, 1, 3} of the interval [-2, 3].
  9. Evaluate:
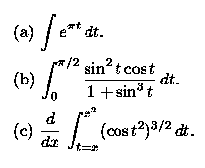
  10. Find the area of the region bounded by the graphs of y = 3x and y = x³ - x.
  
  

• Final Exam, December 1997

  1. Determine whether each limit exists as a real number as infinity or -infinity, or fails to exist. If the limit exists, evaluate it. Give reasons for your answers.
     1. limit as x goes to infinity of (sqrt (1 + 9x²) / x).
     2. limit as h goes to 0 of (sin ((pi/6) + h) - (1/2)) / h.
     3. limit as x goes to 1 of f (x), where f (1) = 0, f (x) = 2x - 6 for x < 1, f (x) = -(x + 1)² for x > 1.
  2. In each case find f' (x).
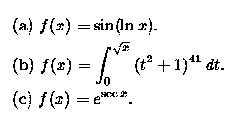
  3. If f (x) = sqrt (x), find f' (4) directly from the definition of the derivative as a limit.
  4. Find an equation of the line tangent to the graph of the equation x² + x²y² + y³ = 13 at the point (1,2).
  5. 1. Explain why x³ + x - 7 = 0 for at least one value x > 0.
     2. Explain why x³ + x - 7 = 0 for at most one value x > 0.
     3. Approximate the value x > 0 with x³ + x - 7 = 0 using the bisection method. Choose the two initial values to be whole numbers and continue until your approximation is within 1/8 of the actual value of x. Show all steps of your calculation.
  6. Find the minimum length of a line segment connecting the point (1,0) to the ellipse given by (x² / 20) + (y² / 15) = 1. Clearly identify the function you are minimizing and the methods you use to find its maximum.
  7. Let f (x) = x² - 8 ln (x² + 3) for all x. Note: f' (x) = 2x - (16 x / x² + 3) and f'' (x) = 2 (x⁴ + 14x² - 14) / (x² + 3)².
     1. What kinds of symmetry, if any, does the graph of f have?
     2. Find the exact values of all critical numbers of the function f.
     3. Determine if f has a relative maximum value, relative minimum value, or neither at each of these critical numbers. Be sure to explain why.
     4. Determine the exact value of the x and y coordinates of each inflection point of f.
  8. A function f is decreasing on [0,2] and increasing on [2,4]. the partition P consists of the points {0, 1/2, 1, 3/2, 2, 5/2, 3, 7/2, 4}. A table of values of f is given below.

     x	0	1/2	1	3/2	2	5/2	3	7/2	4
     f (x)	8	6	5	2	1	2	4	9	10

     
     
     1. Sketch the graph of such a function f defined on [0,4] with these values.
     2. Find the left sum for the function f and the partition P.
     3. Find the lower sum for the function f and the partition P.
  9. A person is flying a kite. The kite is moving with the wind horizontally above the ground at a height of 300 feet. When 500 feet of string have been let out, the kite is pulling string out at a rate of 20 feet per second. Find the wind velocity.
  10. Find the exact value of:
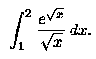
  11. Let f (x) = x³ + x² - 2x - 1 and g (x) = x³ - 1. Find the area A of the bounded region between the graphs of f and g.
  
  

• Final Exam, December 1992

  1. Find the derivative of the given function:
     1. f (x) = (sin 3x) / (1 + x²).
     2. g (x) = x ln (1 + x²).
  2. Find an equation of the line tangent to the graph of x - y² = 2x² - 4y at the point (-1,1).
  3. 1. Evaluate the limit as x -> -3 of (x² - 9) / (x + 3).
     2. Evaluate the limit as x -> 0 of (tan 3x) / (2x).
     3. Using the known inequality u² - (u⁴ / 2) <= ln (1 + u²) <= u² for 0 < |u| < 1, evaluate the limit as u -> 0 of (ln (1 + u²) / u²). Explain your solution.
  4. Sand falling at the rate of 2 cubic inches per second forms a conical pile so that the radius of the base of the cone always equals twice the height of the cone. Find the rate at which the radius is changing at the instant the height is 6 inches. (The volume of a cone with base radius r and height h is pi r²h / 3.)
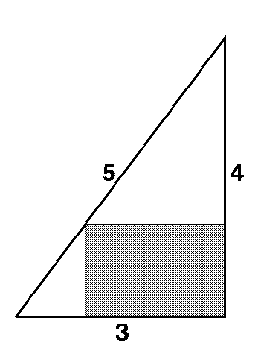
  5. Find the maximum area of a rectangle inscribed in a 3-4-5 right triangle as in the diagram. Check that your answer is the maximum area.
  6. For the first few seconds of a car race, the distance a car has traveled is given by f(t) = t²e^t (feet). Find the velocity of the car after 2 seconds.
  7. Suppose g is a function with second derivative g''(x) = x³(x - 1)²(x - 2)⁵. Find the values of c such that (c, g(c)) is an inflection point of the graph of g.
  8. Let f(x) = x² ln x.
     1. Find the critical point(s) of f.
     2. For each critical point c you found in part (1), determine whether f has a relative maximum, a relative minimum, or neither at c. Explain your answer.
     3. Does f have either a global maximum or a global minimum value on (0, infinity)? Justify your answer.
  9. 
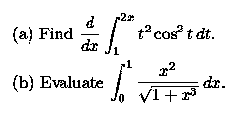
  10. Find the area A of the bounded region between the graphs of y = 2x and y = 2x² - 4x + 4. As part of your solution, indicate clearly which graph forms the upper boundary of the region.
  11. For the last problem you have a choice. Do one or the other of the two parts, but not both. Only one part will be graded.
      1. If you plot y = x⁴ - 3x³ + x - 7 on your calculator, you will see that there are two roots of the equation x⁴ - 3x³ + x - 7 = 0. Use the Newton-Raphson method, starting with an initial guess obtained from your graph, to approximate the larger root. Continue to apply the Newton-Raphson method until the calculator's output no longer changes. Write down only your initial guess and the final answer.
      2. Using the known inequality 2x⁴ <= x⁴(4 + 5x³)^1/2 <= 3x⁴ for 0 <= x <= 1, find positive upper and lower bounds for the integral from 0 to 1 of x⁴(4 + 5x³)^1/2. Show your work. Do not attempt to evaluate the integral exactly.